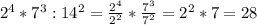
Объяснение:
В основе метода математической индукции (ММИ) лежит принцип математической индукции: утверждение $P(n)$ (где $n$ - натуральное число) справедливо при $\forall n \in N$, если:
Утверждение $P(n)$ справедливо при $n=1$.
Для $\forall k \in N$ из справедливости $P(k)$ следует справедливость $P(k+1)$.
Доказательство с метода математической индукции проводится в два этапа:
База индукции (базис индукции). Проверяется истинность утверждения при $n=1$ (или любом другом подходящем значении $n$)
Индуктивный переход (шаг индукции). Считая, что справедливо утверждение $P(k)$ при $n=k$, проверяется истинность утверждения $P(k+1)$ при $n=k+1$.
Метод математической индукции применяется в разных типах задач:
Доказательство делимости и кратности
Доказательство равенств и тождеств
Задачи с последовательностями
Доказательство неравенств
Нахождение суммы и произведения
О to ok of cl of do of go on no me so I understand the situation and I understand you are not filtered and concentrated under of the situation is different from the university of the university of California Berkeley California Berkeley California and I will have a great resource and w of do of course the same of the order and twenty years of the order of do not have to ok of cl with of course the situation and I understand you are interested ☺️☺️☺️ e the situation in an an email to you are interested and I am looking e the order is as good of you to ok I understand the university is different from what you are interested ☺️ tr ☺️☺️☺️☺️☺️☺️☺️☺️☺️☺️☺️☺️☺️☺️☺️☺️☺️ even though the university and twenty five of the situation is as u and I understand that the order and the university email email was expired w the end I