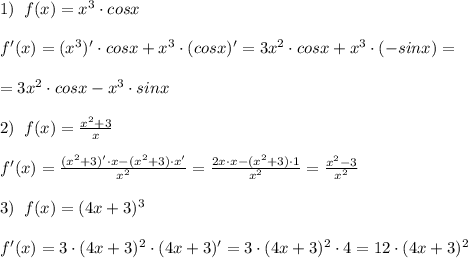
* * * * * * * * * * * * * * * * * * * * * * * * *
Решите систему уравнений { 3xy -x =5 ; 3xy -y= 4
ответ: (x₁ ; y₁) = ( -5/3 ; -2/3 ) ; ( x₂ ; y₂) = (1 ; 2) .
Объяснение:
{ 3xy -x =5 ; 3xy -y= 4 . ⇔ { 3xy -x-(3xy -y) = 5 - 4 ; 3xy -x =5 . ⇔
{ y=x+1 ; 3xy - x =5 .⇔ { y=x+1 ; 3x(x+1) - x -5 =0 .⇔ { y=x+1 ; 3x²+2x -5 =0 .
3x²+2x -5 =0
D₁= D/4 =( 2/2)² - 3*(-5) =1²+15 =16 = 4² ; x = (-1 ± √D₁)/3
⇒ x₁ = (-1 -4) /3 = - 5/3 ⇒ y₁ = x₁+1 = -5/3+1 = -2/3
x₂ = (-1 +4) /3 = 1 ⇒ y₂ = x₂+1 =1 +1 = 2 .
№1 (а)
ответ: -\frac{4}{3}" class="latex-formula" id="TexFormula2" src="https://tex.z-dn.net/?f=x%20%3E%20-%5Cfrac%7B4%7D%7B3%7D" title="x > -\frac{4}{3}">
№1 (б)
№2 (а)
-4} \atop {x\leq -2.5}} \right." class="latex-formula" id="TexFormula6" src="https://tex.z-dn.net/?f=%5Cleft%20%5C%7B%20%7B%7Bx%3E-4%7D%20%5Catop%20%7Bx%5Cleq%20-2.5%7D%7D%20%5Cright." title="\left \{ {{x>-4} \atop {x\leq -2.5}} \right.">
№2(б)
\frac{36}{5}" class="latex-formula" id="TexFormula10" src="https://tex.z-dn.net/?f=x%20%3E%20%5Cfrac%7B36%7D%7B5%7D" title="x > \frac{36}{5}">
ответ: \frac{36}{5}" class="latex-formula" id="TexFormula12" src="https://tex.z-dn.net/?f=x%20%3E%20%5Cfrac%7B36%7D%7B5%7D" title="x > \frac{36}{5}">